It’s May 1964 and, on a low hillside in New Jersey, the physicists Robert Woodrow Wilson and Arno Allan Penzias are listening in on the Universe. They are standing beneath what looks like a gargantuan ear trumpet attached to a garden shed: the Holmdel Horn Antenna, built by Bell Laboratories to investigate microwaves as an alternative to radio waves for telecommunication. When interest in microwave communication waned, Bell lent out the Holmdel horn to interested scientists.
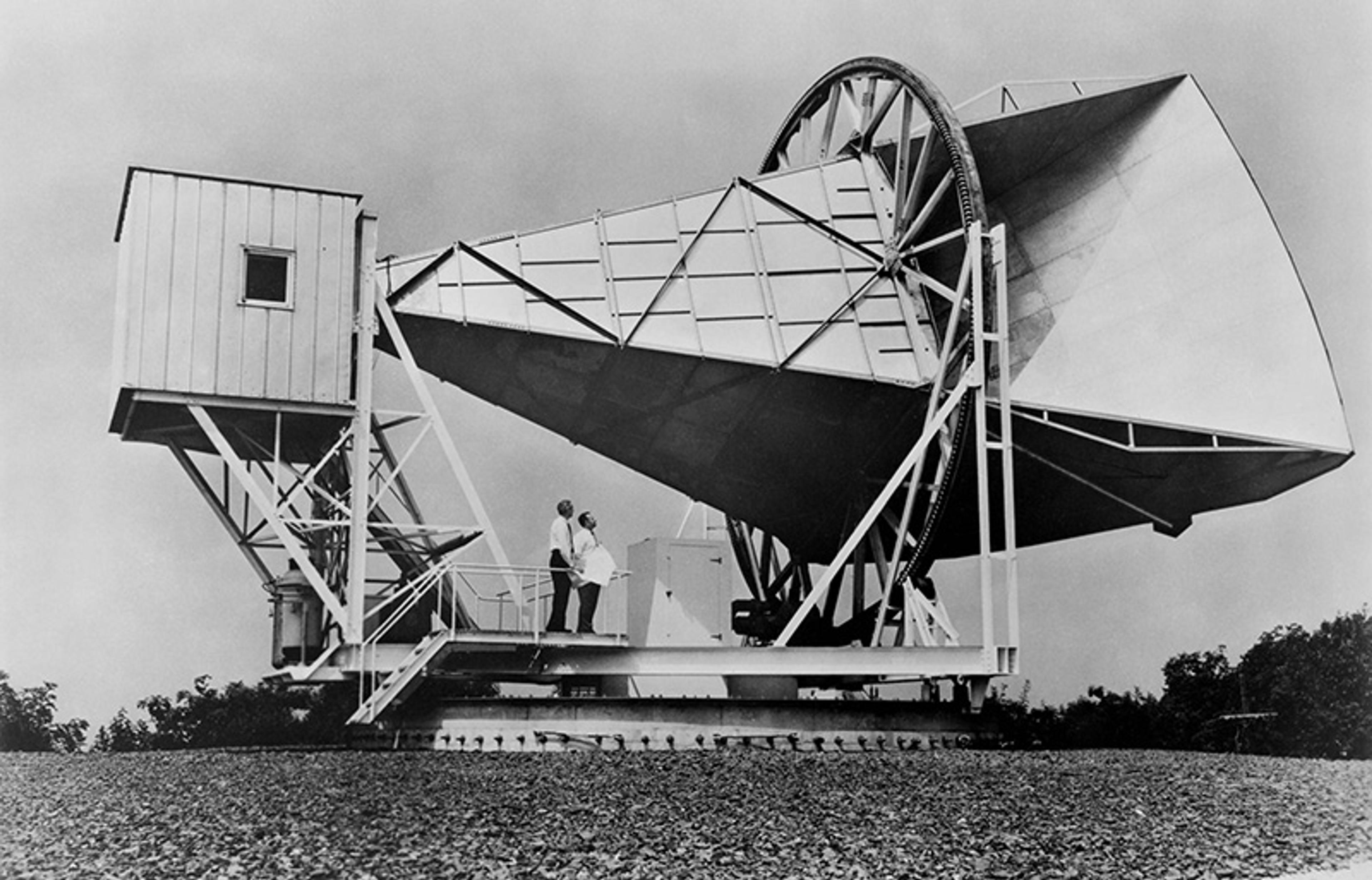
Penzias and Wilson were interested. Both aged around 30, they planned to map the sky with microwaves. But they were baffled: when they pointed the horn at a dark region beyond the galaxy and only sparsely populated with stars, instead of the silence they expected, they detected a kind of background hiss – a hiss that filled the entire sky.
Meanwhile, the physicist Robert H Dicke was working on a related puzzle. Two decades earlier, Dicke had invented the microwave detector. Now he and his lab were trying to develop sensitive instruments to test the cosmological predictions that emerged from Albert Einstein’s general theory of relativity, particularly how it related to Edwin Hubble’s astonishing discovery that the Universe is expanding. The reigning, steady-state theory claimed that the Universe had always been expanding, balanced by a continuous creation of new matter. The rival theorists, including Dicke, took expansion at its face value, running it backwards in time to propose that, about 14 billion years ago, the Universe burst into existence in a cataclysmic explosion from a very tiny point.
An exploding universe should have left a uniform faint cloud of microwave radiation, which Dicke’s team was determined to find. News of the group’s efforts reached Penzias and Wilson, prompting Penzias to give Dicke a call. Over a brownbag lunch, Dicke’s colleagues recall him picking up the receiver, repeating phrases such as ‘horn antenna’ and nodding. After hanging up, he turned to his group and said: ‘Well boys, we’ve been scooped.’ Dicke realised that Penzias and Wilson had discovered the Big Bang.
The uniformity of the cosmic microwave background (CMB) tells us that, at its birth, ‘the Universe has turned out to be stunningly simple,’ as Neil Turok, director emeritus of the Perimeter Institute for Theoretical Physics in Ontario, Canada, put it at a public lecture in 2015. ‘[W]e don’t understand how nature got away with it,’ he added. A few decades after Penzias and Wilson’s discovery, NASA’s Cosmic Background Explorer satellite measured faint ripples in the CMB, with variations in radiation intensity of less than one part in 100,000. That’s a lot less than the variation in whiteness you’d see in the cleanest, whitest sheet of paper you’ve ever seen.
Wind forward 13.8 billion years, and, with its trillions of galaxies and zillions of stars and planets, the Universe is far from simple. On at least one planet, it has even managed to generate a multitude of life forms capable of comprehending both the complexity of our Universe and the puzzle of its simple origins. Yet, despite being so rich in complexity, some of these life forms, particularly those we now call scientists, retain a fondness for that defining characteristic of our primitive Universe: simplicity.
The Franciscan friar William of Occam (1285-1347) wasn’t the first to express a preference for simplicity, though he’s most associated with its implications for reason. The principle known as Occam’s Razor insists that, given several accounts of a problem, we should choose the simplest. The razor ‘shaves off’ unnecessary explanations, and is often expressed in the form ‘entities should not be multiplied beyond necessity’. So, if you pass a house and hear barking and purring, then you should think a dog and a cat are the family pets, rather than a dog, a cat and a rabbit. Of course, a bunny might also be enjoying the family’s hospitality, but the existing data provides no support for the more complex model. Occam’s Razor says that we should keep models, theories or explanations simple until proven otherwise – in this case, perhaps until sighting a fluffy tail through the window.
Seven hundred years ago, William of Occam used his razor to dismantle medieval science or metaphysics. In subsequent centuries, the great scientists of the early modern era used it to forge modern science. The mathematician Claudius Ptolemy’s (c100-170 CE) system for calculating the motions of the planets, based on the idea that the Earth was at the centre, was a theory of byzantine complexity. So, when Copernicus (1473-1543) was confronted by it, he searched for a solution that ‘could be solved with fewer and much simpler constructions’. The solution he discovered – or rediscovered, as it had been proposed in ancient Greece by Aristarchus of Samos, but then dismissed by Aristotle – was of course the solar system, in which the planets orbit around the Sun. Yet, in Copernicus’s hands, it was no more accurate than Ptolemy’s geocentric system. Copernicus’s only argument in favour of heliocentricity was that it was simpler.
Nearly all the great scientists who followed Copernicus retained Occam’s preference for simple solutions. In the 1500s, Leonardo da Vinci insisted that human ingenuity ‘will never devise any [solutions] more beautiful, nor more simple, nor more to the purpose than Nature does’. A century or so later, his countryman Galileo claimed that ‘facts which at first seem improbable will, even on scant explanation, drop the cloak which has hidden them and stand forth in naked and simple beauty.’ Isaac Newton noted in his Principia (1687) that ‘we are to admit no more causes of natural things than such as are both true and sufficient to explain their appearances’; while in the 20th century Einstein is said to have advised that ‘Everything should be made as simple as possible, but not simpler.’ In a Universe seemingly so saturated with complexity, what work does simplicity do for us?
Simple scientific laws are preferred because, if they fit or fully explain the data, they’re more likely to be the source of it
Part of the answer is that simplicity is the defining feature of science. Alchemists were great experimenters, astrologers can do maths, and philosophers are great at logic. But only science insists on simplicity. Many advances of modern science involved a succession of simplifications, either through unifying previously disparate phenomena or by eliminating superfluous entities. Probably the greatest simplification was provided by Newton, who unified trillions of motions, both on Earth and in the heavens, into just three laws of motion and one of gravity. Then in the late 19th century, Ludwig Boltzmann extended Newton’s laws into the microscopic realm to provide an economical explanation of heat as a measure of the motion of atoms. Einstein achieved perhaps the most radical simplification by unifying space and time within a single entity, spacetime. Charles Darwin and Alfred Russel Wallace had brought the entire natural world under a single law of natural selection; while the work of Louis Pasteur, Gregor Mendel, Hugo de Vries, James Watson, Francis Crick and many others dispensed with the vital principle to extend simple scientific laws into biology. Each scientist considered their advance to deliver a simplification that eliminated superfluous complexity. As Wallace, the co-discover of natural selection, put it: ‘The theory itself is exceedingly simple.’

Just why do simpler laws work so well? The statistical approach known as Bayesian inference, after the English statistician Thomas Bayes (1702-61), can help explain simplicity’s power. Bayesian inference allows us to update our degree of belief in an explanation, theory or model based on its ability to predict data. To grasp this, imagine you have a friend who has two dice. The first is a simple six-sided cube, and the second is more complex, with 60 sides that can throw 60 different numbers. Suppose your friend throws one of the dice in secret and calls out a number, say 5. She asks you to guess which dice was thrown. Like astronomical data that either the geocentric or heliocentric system could account for, the number 5 could have been thrown by either dice. Are they equally likely? Bayesian inference says no, because it weights alternative models – the six- vs the 60-sided dice – according to the likelihood that they would have generated the data. There is a one-in-six chance of a six-sided dice throwing a 5, whereas only a one-in-60 chance of the 60-sided dice throwing a 5. Comparing likelihoods, then, the six-sided dice is 10 times more likely to be the source of the data than the 60-sided dice.
Simple scientific laws are preferred, then, because, if they fit or fully explain the data, they’re more likely to be the source of it. With more knobs to tweak, arbitrarily complex models such as Ptolemy’s astronomical system could be made to fit any dataset. As the mathematician John von Neumann once quipped: ‘with four parameters I can fit an elephant, and with five I can make him wiggle his trunk’.
Is there more to simplicity than probability? Many of the greatest scientists and philosophers were devotees of what might be called a strong version of Occam’s Razor. This claims that the world is about as simple as it can be, consistent with our existence. The theoretical physicist and Nobel Laureate Eugene Wigner’s influential paper ‘The Unreasonable Effectiveness of Mathematics in the Natural Sciences’ (1960) argued that the extraordinary ability of mathematics to make sense of the world is a puzzle. An analogous case can be made for the success of simplicity in science. Why is Occam’s Razor so unreasonably effective? Why does simplicity work so well?
Consider how, when Einstein first attempted to incorporate gravity and acceleration into relativity, he eschewed any considerations of ‘beauty and simplicity’. Instead, he favoured what is called completeness: the incorporation of the maximum amount of available information into a model. Yet a decade of struggling with complex equations met with failure. He eventually changed tack to embrace Occam’s Razor, accepting only the simplest and most elegant equations, and later testing them against physical facts. This time, Einstein struck gold, unearthing his general theory of relativity in 1915. Thereafter, he insisted that ‘equations of such complexity … can be found only through the discovery of a logically simple mathematical condition that determines the equations completely or almost completely.’
But could it be simpler still? Why are there 17 particles in the Standard Model of particle physics when we are composed of only a handful? If the Universe is maximally simple, why are trillions of almost massless and electrically neutral neutrinos passing through our bodies every second? Surely neutrinos are entities beyond our necessity? Another candidate for an entity beyond necessity is the mysterious dark matter, of which our Universe appears to be chiefly composed. Why does a simple universe harbour so much apparently superfluous stuff?
In fact, both dark matter and neutrinos are essential for our existence. Neutrinos are a necessary byproduct of the stellar nuclear-fusion reactions that fuse protons to make helium nuclei, plus the heat and light that make life possible. One of physics’ laws of conservation demands that the total number of leptons (electrons, muons, tau particles and neutrinos) must remain constant. This can be satisfied in the stellar fusion reaction only through the release of massive numbers of neutrinos. Similarly for dark matter. In the early Universe, it acted as a kind of cosmological clotting agent that helped to coalesce the diffuse gas that emerged from the Big Bang into the lumpy clouds that became galaxies, stars, planets and eventually us. Haloes of dark matter at the edge of galaxies also act as galactic guardians, deflecting high-speed supernovae remnants rich in the heavy elements essential for life, from shooting off into the vast empty reaches of intergalactic space.
In my latest book, I propose a radical, if speculative, solution for why the Universe might in fact be as simple as it’s possible to be. Its starting point is the remarkable theory of cosmological natural selection (CNS) proposed by the physicist Lee Smolin. CNS proposes that, just like living creatures, universes have evolved through a cosmological process, analogous to natural selection.
The process of mutational pruning of inessential functions is a kind of evolutionary Occam’s Razor
Smolin came up with CNS as a potential solution to what’s called the fine-tuning problem: how the fundamental constants and parameters, such as the masses of the fundamental particles or the charge of an electron, got to be the precise values needed for the creation of matter, stars, planets and life. CNS first notes the apparent symmetry between the Big Bang, in which stars and particles were spewed out of a dimensionless point at the birth of our Universe, and the Big Crunch, the scenario for the end of our Universe when a supermassive black hole swallows up stars and particles before vanishing back into a dimensionless point. This symmetry has led many cosmologists to propose that black holes in our Universe might be the ‘other side’ of Big Bangs of other universes, expanding elsewhere. In this scenario, time did not begin at the Big Bang, but continues backwards through to the death of its parent universe in a Big Crunch, through to its birth from a black hole, and so on, stretching backward in time, potentially into infinity. Not only that but, since our region of the Universe is filled with an estimated 100 billion supermassive black holes, Smolin proposes that each is the progenitor of one of 100 billion universes that have descended from our own.
The model Smolin proposed includes a kind of universal self-replication process, with black holes acting as reproductive cells. The next ingredient is heredity. Smolin proposes that each offspring universe inherits almost the same fundamental constants of its parent. The ‘almost’ is there because Smolin suggests that, in a process analogous to mutation, their values are tweaked as they pass through a black hole, so baby universes become slightly different from their parent. Lastly, he imagines a kind of cosmological ecosystem in which universes compete for matter and energy. Gradually, over a great many cosmological generations, the multiverse of universes would become dominated by the fittest and most fecund universes, through their possession of those rare values of the fundamental constants that maximise black holes, and thereby generate the maximum number of descendant universes.
Smolin’s CNS theory explains why our Universe is finely tuned to make many black holes, but it does not account for why it is simple. I have my own explanation of this, though Smolin himself is not convinced. First, I point out that natural selection carries its own Occam’s Razor that removes redundant biological features through the inevitability of mutations. While most mutations are harmless, those that impair vital functions are normally removed from the gene pool because the individuals carrying them leave fewer descendants. This process of ‘purifying selection’, as it’s known, maintains our genes, and the functions they encode, in good shape.
However, if an essential function becomes redundant, perhaps by a change of environment, then purifying selection no longer works. For example, by standing upright, our ancestors lifted their noses off the ground, so their sense of smell became less important. This means that mutations could afford to accumulate in the newly dispensable genes, until the functions they encoded were lost. For us, hundreds of smell genes accumulated mutations, so that we lost the ability to detect hundreds of odours that we no longer need to smell. This inevitable process of mutational pruning of inessential functions provides a kind of evolutionary Occam’s Razor that removes superfluous biological complexity.
Perhaps a similar process of purifying selection operates in cosmological natural selection to keep things simple. Instead of biological mutations, we have tweaks to fundamental constants of universes as they pass through black holes. Let’s imagine that our Universe contains two black holes that are the proud parents of two baby universes. When the constants (the masses of particles, the charge of an electron and so forth) pass through the first black hole, they remain unchanged. As a consequence, a universe very like our own evolves, which we will call the 17P universe, reflecting the fact that it possesses 17 fundamental particles. However, in the second black hole, a tweak (mutation) to the fundamental constants generates a universe with one extra particle. This particle plays no role in black-hole formation, nor in the formation of stars or life, but instead merely hangs around, perhaps in intergalactic clouds. The 18th particle is an entity beyond necessity in this 18P universe.
Let’s additionally suppose that 18P’s extra particle has average mass and abundance for fundamental particles, so that it accounts for about one-18th of total mass in that universe. This locking away of mass in the intergalactic clouds of particle 18 will diminish the amount of matter/energy available for black-hole formation. As a consequence, the presence of particle 18 will reduce the number of black holes by one-18th, or about 5 per cent. Since black holes are the mothers of universes, the 18P universe will generate around 5 per cent fewer progeny than its sibling, the 17P universe. This difference in fecundity will continue into succeeding generations until, by about generation 20, descendants of the 18P universe will be one-third as abundant as descendants of the more parsimonious 17P universe. In the natural world, mutations that lead to just a 1 per cent reduction in fitness are sufficient to drive a mutant into extinction, so a 5 per cent decrease in fitness is likely to eliminate, or at least drastically reduce, the abundance of 18-particle universes, relative to their more parsimonious 17-particle universes.
It’s unclear whether the kind of multiverse envisaged by Smolin’s theory is finite or infinite. If infinite, then the simplest universe capable of forming black holes will be infinitely more abundant than the next simplest universe. If instead the supply of universes is finite, then we have a similar situation to biological evolution on Earth. Universes will compete for available resources – matter and energy – and the simplest that convert more of their mass into black holes will leave the most descendants. For both scenarios, if we ask which universe we are most likely to inhabit, it will be the simplest, as they are the most abundant. When inhabitants of these universes peer into the heavens to discover their cosmic microwave background and perceive its incredible smoothness, they, like Turok, will remain baffled at how their universe has managed to do so much from such a ‘stunningly simple’ beginning.
The cosmological razor idea has one further startling implication. It suggests that the fundamental law of the Universe is not quantum mechanics, or general relativity or even the laws of mathematics. It is the law of natural selection discovered by Darwin and Wallace. As the philosopher Daniel Dennett insisted, it is ‘The single best idea anyone has ever had.’ It might also be the simplest idea that any universe has ever had.






